
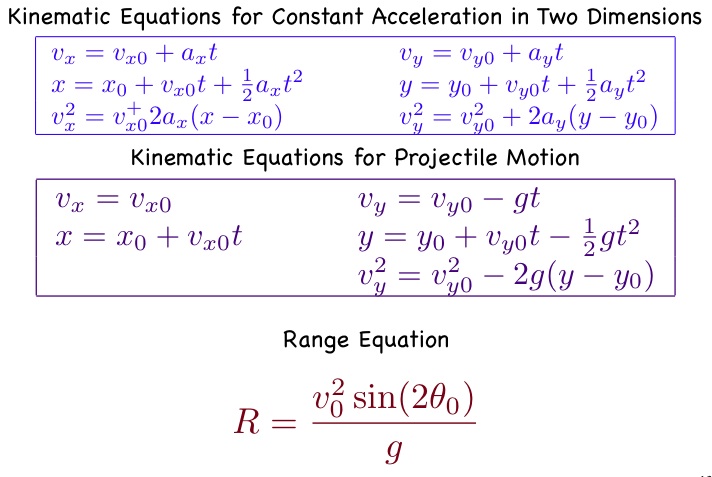
Now we will investigate the manner in which the horizontal and vertical components of a projectile's displacement vary with time. Thus the range of the projectile depends upon the velocity of projection and the angle of projection.The previous diagrams, tables, and discussion pertain to how the horizontal and vertical components of the velocity vector change with time during the course of projectile's trajectory. To determine the range R of the projectile, we multiply the horizontal component of the velocity of projection with the total time taken by the body after leaving the point of projection. Or The horizontal distance covered by the projectile between the point of projection and point of return to the level of projection is called the “Range” of the projectile and is represented by “R”. The maximum distance that a projectile covers in the horizontal direction is called the range of the projectile. If the body is projecting with velocity V making angle θ with a horizontal, then its vertical component will be Visinθ. This can be obtained by taking S =h =0, because the body goes up and comes back to the same level, thus covering no vertical distance. “It is the total time for which the projectile remains in the air.” or The time taken by the body to cover the distance from the place of its projection to the place where it hits the ground at the same level is called the time of flight.

It is denoted by H.Īs the body moves upward, so a = -g, the initial vertical velocity V iy =V i sinθ and V fy =0 because the body comes to rest after reaching the highest point. The maximum vertical distance attained by the projectile is called maximum height. In P motion one may wish to determine the height to which the projectile rises, the time of flight and horizontal range. The angle Φ which this resultant velocity makes with the horizontal can be found from: The magnitude of velocity at any instant is:

By using the first equation of motion (V f = V i + at) the vertical component V fy of the velocity at any instant t is given by: The initial vertical component of the velocity is V sinθ in the upward direction. Hence, the horizontal component V x remains constant and at any time t, we have: The horizontal acceleration is ax = 0 because we have neglected air resistance and no other force is acting along with the direction resistance and no other force is acting along this direction whereas vertical acceleration a y = g. Suppose that a projectile is fired in a direction angle θ with the horizontal by velocity V along the horizontal and vertical components of velocity V along the horizontal and vertical directions be V cosθ and V sinθ respectively.

In such cases, the motion of a projectile can be studied easily by resolving it into horizontal and vertical components that are independent of each other. A football kicked off by a player a ball thrown by a cricketer and a missile fired from a launching pad, all projected at some angles with the horizontal, are called projectiles. It is not necessary that an object should be thrown with some initial velocity in the horizontal direction. Since initial velocity is zero, hence, vertical distance y by using the second equation of motion is given by: This vertical motion is the same as for a freely falling body. It will accelerate downward under the force of gravity and hence a = g. The vertical motion of the ball is also not complicated.


 0 kommentar(er)
0 kommentar(er)
